CONTENT LIST
Introduction
Quadrilateral word is derived from the Latin words 'quadri,'which means four, and 'latus', which means side.
A quadrilateral is a polygon of four sides, four angles, and four vertices.
Cards, chess boards, traffic signs, etc. are real life objects of quadrilateral shape.
Parts of a quadrilateral
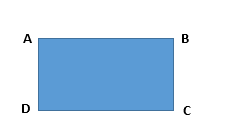
- Four angles of quadrilateral ABCD are ∠A, ∠B, ∠C, and ∠D .
- Four sides of quadrilateral ABCD are AB, BC, CD, and DA.
- Four vertex of quadrilateral ABCD are A, B, C, and D.
- Two diagonals of quadrilateral ABCD are AC and BD .
Angle Sum Property of a Quadrilateral
The sum of the four angles of a quadrilateral is 360°
ABCD is a quadrilateral then
∠A+∠B+∠C+ ∠D=360°
Types of Quadrilaterals
Quadrilaterals can be classified into categories that are Parallelograms, Squares, Rectangles, Trapezium Rhombuses and Kite.
Parallelogram: A quadrilateral with both pairs of opposite sides parallel is called parallelograms.
Square: A parallelogram having all sides equal and one angle at a right angle is called square.
Rectangle: A parallelogram with one angle at a right angle is called a rectangle.
Trapezium: A quadrilateral with at least one pair of opposite sides parallel is called trapezium.
Rhombus: A parallelogram having all sides equal is called rhombus.
Kite: A quadrilateral with two pairs of adjacent sides equal, but opposite sides are not parallel, is kite.
Concave & Convex quadrilaterals
Concave quadrilaterals: In concave quadrilaterals, any one interior angle is greater than 180°.
The line segment joining the vertices is not a part of the same region of the quadrilateral.
Convex quadrilaterals: In convex quadrilaterals, each interior angle is less than 180°.
A quadrilateral is convex if the line segment joining any of its two vertices is in the same region.
Properties of a Parallelogram
Theorem : A diagonal of a parallelogram divides it into two congruent triangles.
Theorem : In a parallelogram, opposite sides are equal.
Theorem : If each pair of opposite sides of a quadrilateral is equal, then it
is a parallelogram.Theorem : In a parallelogram, opposite angles are equal.
Theorem : The diagonals of a parallelogram
bisect each other.Theorem : If the diagonals of a quadrilateral bisect each other, then it is a parallelogram.
Theorem : A quadrilateral is a parallelogram if a pair of opposite sides is
equal and parallel.Condition for a Quadrilateral to be a Parallelogram
(i) Each pair of opposite sides are parallel.
(ii) Each pair of opposite sides are equal.
(iii) Each pair of opposite angles are equal.
(iv) Diagonals bisect each other.
(v) One pair of opposite sides are parallel and equal.
The Mid-point Theorem
A line segment joining the midpoint of any two sides of the triangle is parallel to its third side and is also half of the length of the third side.
Converse of Mid-Point Theorem
The line drawn through the mid-point of one side of a triangle parallel to the base of a triangle bisects the third side of the triangle.
Related Topics